I’ve even got a starter question to get you guys into the scenario.
Once you’ve completed the starter question, under the solution comment attaches the main question, which is unsolved.
Kind-of solution
F = ma and m = 1, so a = F = 1/r^2. I used a = -1/r^2 so the force experienced by the particle at r = 5 would be negative. a = dv/dt.
dv/dt = -1/r^2
v dv/dt = -1/r^2 dr/dt → v = dr/dt. Multiply both sides by v, but write it as dr/dt on the right
v dv = -1/r^2 dr → Multiply both sides by dt
0.5v^2 = 1/r + C → Integrate
v^2 = 2/r + C → Multiply both sides by 2, find C = -2/5 by plugging in r = 5, v = 0
v^2 = 2/r - 2/5
5*arcsin(sqrt(r/5)) - sqrt(5r-r^2) = sqrt(2/5) t + C → Solve the differential equation y’^2 = 2/y - 2/5 with Wolfram Alpha, find C = 5π/2 by plugging in r = 5, t = 0
5*arcsin(sqrt(r/5)) - sqrt(5r-r^2) = sqrt(2/5) t + 5π/2 → Can find that the particle reaches the origin at t = 5sqrt(5)π / 2sqrt(2) ≈ 12.418 seconds
I made heavy use of internet resources on this one, not just for solving the differential equation but also for technique - I got a bunch of wrong answers before getting this one that I finally think is right, at least up until the moment of origin contact
Graph (the hidden ellipse is because I thought the path may be elliptical, but it doesn’t appear to be)
Solution (starter question):
spoiler
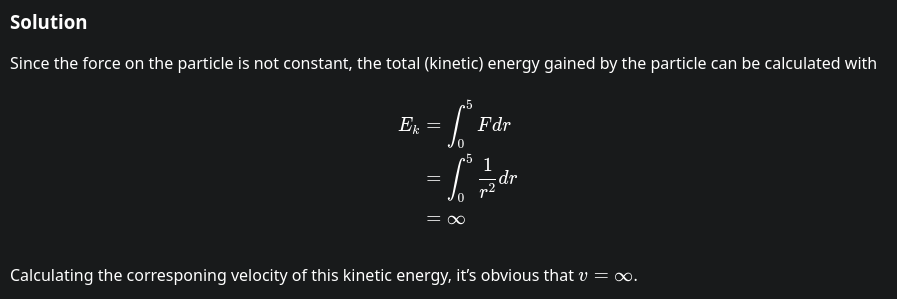
Please refer to the main post, if you don’t like looking at the image. https://gmtex.siri.sh/fs/1/School/Extra/Maths/Unsolved/1d-gravity.html
For the main question, you are encouraged to share your progress
spoiler

You might be able to solve this with differential equations, or by solving the iterative functions, I dont know
Not sure how much you’d care, but I came back to this and found (by hand) a function which closely approximates the solution - it’s not exact but it’s also not super far. Graph.
I think I could also solve the differential equation by hand at this point (getting the same solution as before) - I haven’t, but I’m pretty sure I could if I wanted to for whatever reason. I’m doubtful it’s possible to get an exact solution in terms of just x but if you ever manage I’d love to see how.